Stable Manifold Theorem
From Physiki
| Main Page | > | Bill:Lee | > | Mathematics |
Let E be an open subset of 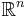 , which contains the origin, let
, which contains the origin, let 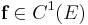 , and let φt be the flow of the nonliear system
, and let φt be the flow of the nonliear system  . Suppose
. Suppose 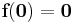 and that
and that 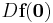 has k eigenvalues with negative real part and n − k eigenvalues with positive real part. Then there exists a k − dimensional differentiable manifold S tangent to the sable subspace Es of the linear system
has k eigenvalues with negative real part and n − k eigenvalues with positive real part. Then there exists a k − dimensional differentiable manifold S tangent to the sable subspace Es of the linear system 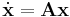 at
at  such that for all
such that for all 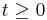 ,
, 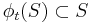 and for all
and for all  ,
, 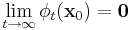 ; and there exists an n − k dimensional differentiable manifold U tangent to the unstable subspace Euof the linear system at
; and there exists an n − k dimensional differentiable manifold U tangent to the unstable subspace Euof the linear system at  such that for all
such that for all 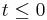 ,
, 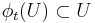 and for all
and for all  ,
, 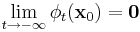
References
Differential Equations and Dynamical Systems : Lawrence Perko, page 109, theorem 2, third edition