Modern 2:More on Square Wells and Review for Monday's Exam

Course Wikis

>

Physics Course Wikis

>

Modern 2

Before starting the review, I would like to go back and redo the infinite square well potential but with a slight variation.

Download a trivial Mathematica notebook with some plots of the results

review

There is a review of important topics at the end of chapter 3, pages 58-59. I will go over this in class today. But briefly, it includes the time dependent and time independent Schrodinger equations. Momentum space representation. The Heisenberg uncertainty relation (for x, p_x and E, t). The idea that to each physical quantity A there is an associated observable given as a self-adjoint operator 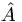$\hat{A}$. And that the expected value of a measurement associated with this quantity is given by $\langle \psi |\hat{A}|\psi \rangle = \int \psi^*(\mathbf{r},t) \left[ \hat{A} \psi(\mathbf{r},t) \right] d^3 r$.

In addition to this summary, you should understand

• the infinite square well sufficiently to be able to derive the energy eigenstates, the energy levels and normalization.

• the eigenstates are orthogonal and complete. This lets us represent any wavefunction as a superposition of eigenstates.

• if the wavefunction IS an eigenfunction of some observable 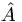$\hat{A}$ with eigenvalue a, the the measurement of A is equal to a with probability 1.

• it would be good to refresh your memory on Fourier series, in case I ask you to represent a non-eigenstate in terms of a superposition of eigenstates. E.g., a Gaussian.

There are two potential problems that will be fair game for Monday's exam. First is the finite step potential:

$V(x) = V_0 \hbox{  if  } 0 \leq x \leq a$ and zero otherwise

$V(x) = 0 \hbox{  if  } 0 \leq x \leq a$ and infinite otherwise

A first order expectation would be that you can set up the solutions in the different regions (knowing under what circumstances you would get a propagating wave and under what you would get an exponentially damped (tunneling) state. Then you should be able to apply any boundary conditions.

I don't expect you to be able to derive the probability current (at least on an exam) but you should know how to compute it and you should understand it's physical significance.