Classical vs quantum harmonic oscillator. Correspondance principle

(Difference between revisions)
Jump to: navigation, search
(→Brief digression, the astounding cancelation properties of Hermite polynomials)
(→Classical versus Quantum harmonic oscillator)
Line 37: Line 37:
 
===Classical versus Quantum harmonic oscillator ===
 
===Classical versus Quantum harmonic oscillator ===
  
 +
We've seen two ways to write the equations of motion for the classical SHO:
  
−
Here are
+
Newtonian:  <math> m \ddot{x} = - k x </math> (assuming the equilibrium position is 0)
 +
 
 +
Hamiltonian:  <math> H,p = \dot{x}  \hspace{5mm} -H,x = \dot{p} </math>
 +
 
 +
where  <math> H,p \equiv \frac{\partial H}{\partial p} </math>
 +
 
 +
For the classical harmonic oscillator, the

Revision as of 15:21, 31 March 2006

some key ideas on the harmonic oscillator

  • ω / is the natural frequency of the oscillator. This

is a property of the potential. Remember, for small displacements from equilibrium: x0.

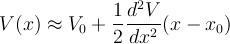
V(x) \approx V_0 + \frac{1}{2}\frac{d^2 V}{dx^2} (x - x_0)

Hence the force associatd with the potential is:

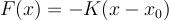
F(x) = - K (x - x_0) \

where 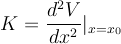K = \frac{d^2 V}{dx^2} |_{x=x_0}

  • in 1D the QHO has an infinite series of equally spaced energy levels:

E_n = (n + 1/2) \hbar \omega

  • many of the calculations become simpler if we introduce dimensionless

variables

\tilde{x} \equiv x \sqrt{m \omega/\hbar}

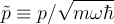
\tilde{p} \equiv p /\sqrt{m \omega \hbar}

In particular, you can show that:

Variance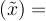( \tilde{x}) = Variance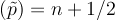( \tilde{p}) = n + 1/2

This is a striking result since it says that for energy eigenstates, nature will not allow us to sacrafice knowledge of one variable, in order to gain knowledge of the other.

Brief digression, the astounding cancelation properties of Hermite polynomials

Mathematica.png Download try this yourself

Classical versus Quantum harmonic oscillator

We've seen two ways to write the equations of motion for the classical SHO: 

Newtonian:   m \ddot{x} = - k x  (assuming the equilibrium position is 0)

Hamiltonian:  Failed to parse (Cannot write to or create math temp directory):  H,p = \dot{x}  \hspace{5mm} -H,x = \dot{p}


where 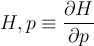H,p \equiv \frac{\partial H}{\partial p}

For the classical harmonic oscillator, the

Retrieved from "http://inksurvey.org/csm/wiki/index.php?title=Classical_vs_quantum_harmonic_oscillator._Correspondance_principle&oldid=798"
Personal tools
Namespaces
Variants
Actions
Navigation
Toolbox